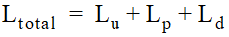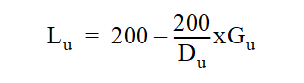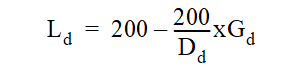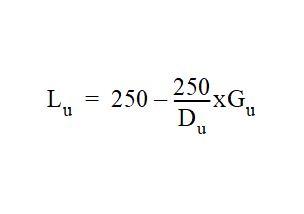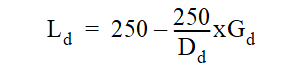Anchor: #CHDCCHCH

Section 6: Determining Length of Need of Barrier

Anchor: #i1005497

Overview

The shape of the obstacle, its location with respect to travel lanes, the volume of traffic and its corresponding clear zone width are the primary variables influencing length of barrier need. Barrier can be rigid such as a concrete barrier, or semi-rigid, such as metal beam guard fence.

Anchor: #i1005508

Variables

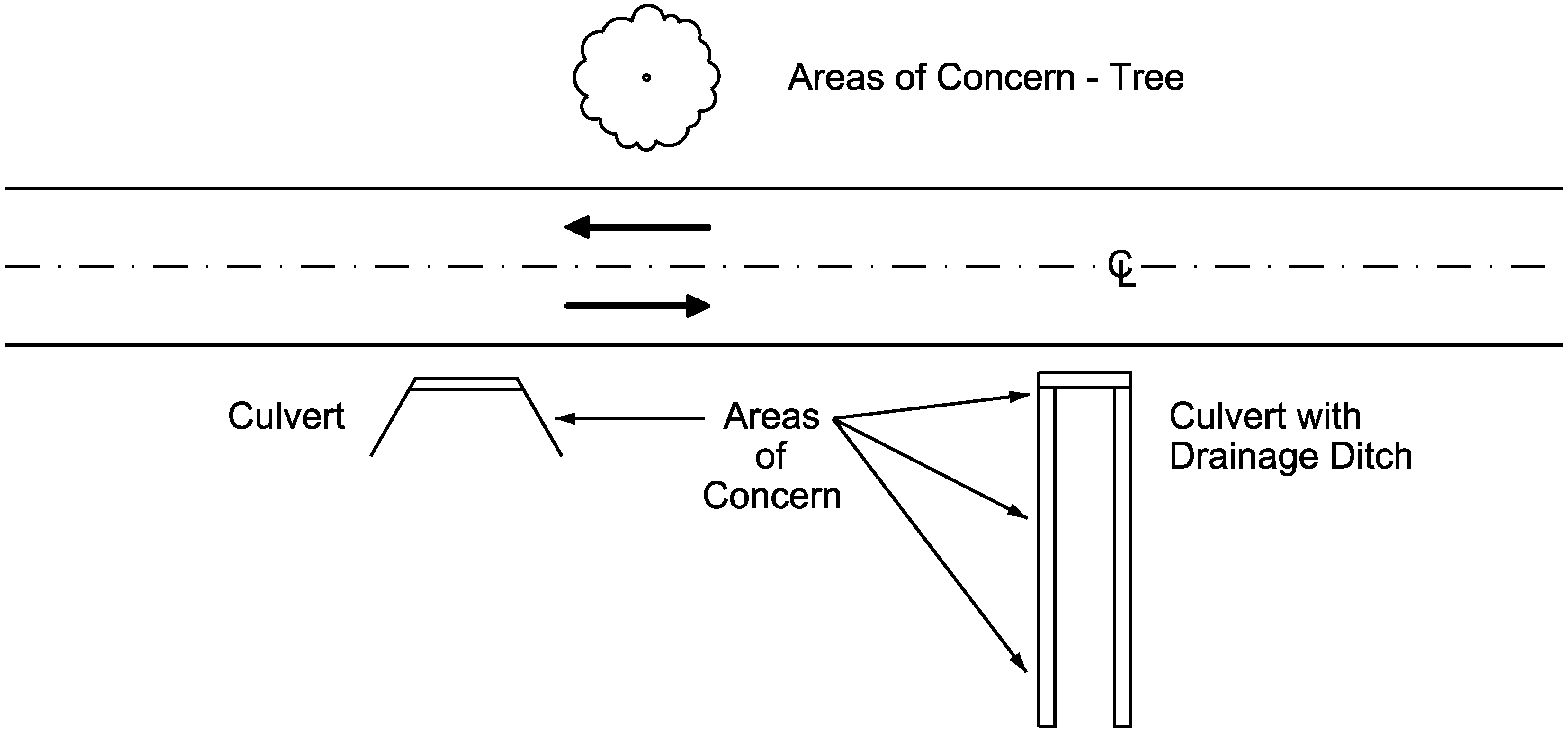
After all practical means to free the roadside of obstacles have been exhausted, certain areas may remain which constitute an obstacle to errant vehicles. These areas, as illustrated in Figure A-7, will be referred to as an “area of concern.”

Areas of Concern (click in image to see full-size image) Anchor: #i1001814grtop

Figure A-7. Areas of Concern

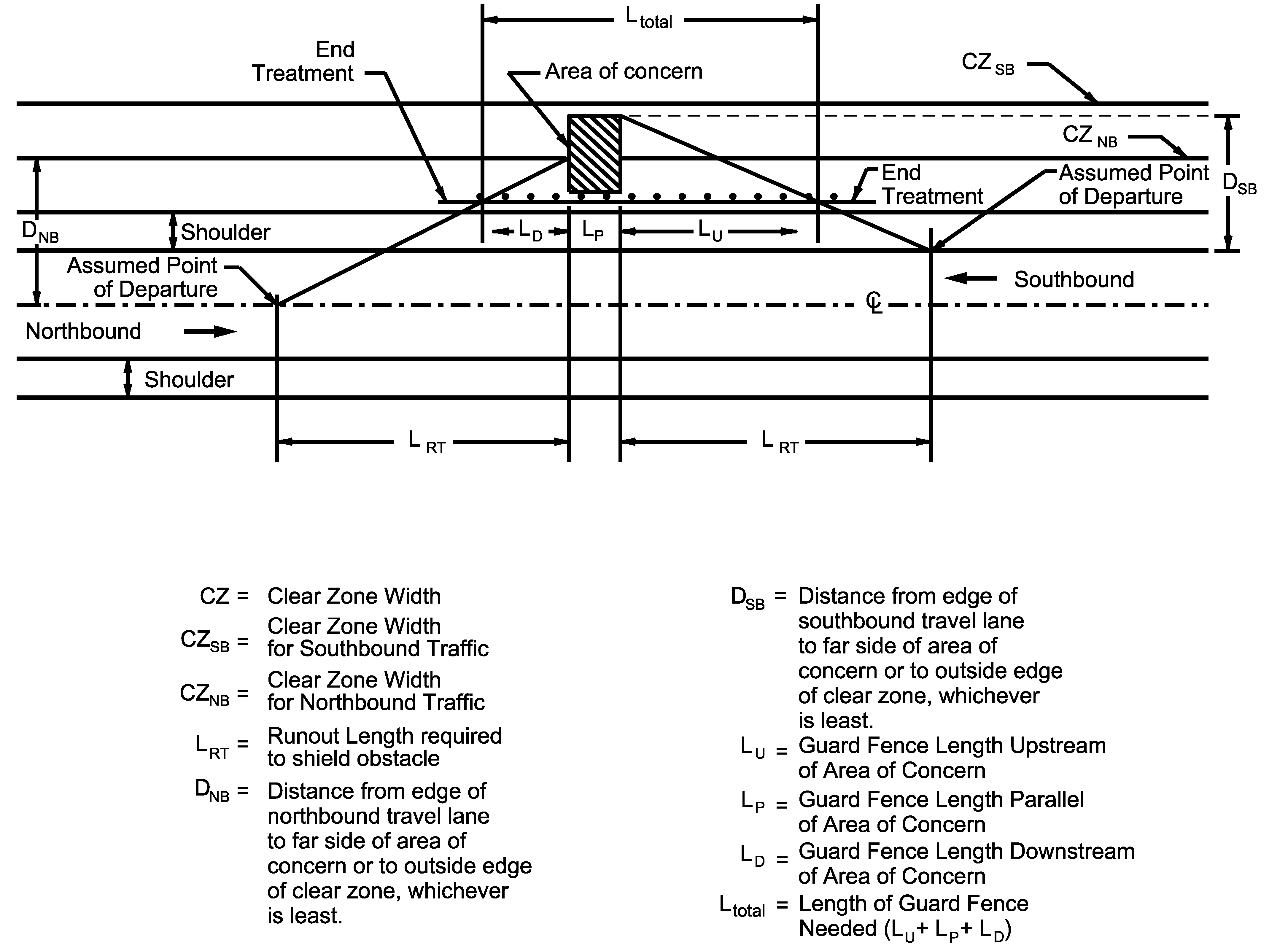
Figure A-8 illustrates the variables of interest in the layout of approach barrier to shield an area of concern. The total length of need is equal to the sum of the following variables:

Anchor: #DVIULFAF

Equation A-1.

Where:

When discussing length of need as it pertains to metal beam guard fence, Lu is the length of guard fence needed to shield the area of concern for adjacent traffic. Upstream refers to the guard fence upstream of traffic adjacent to proposed guard fence. Ld is the length of guard fence needed to protect the opposing traffic. For roadways serving one-way traffic operations, Ld = 0. Ld is greater than zero for two-way operations when the area of concern lies within the clear zone of opposing (northbound in Figure A-8) traffic as measured from the centerline pavement markings.

Variables Involved in Barrier Layout. (click in image to see full-size image) Anchor: #i1001816grtop

Figure A-8. Variables Involved in Barrier Layout.

In certain instances, judgment should be exercised to supplement design chart solutions and provide for additional safety. For example, high severity fixed objects (e.g., bridge columns) may justify minimum guard fence treatment where located outside the clear zone if geometric conditions (i.e., steep fill slope, outside of horizontal curvature, etc.) increase the likelihood of roadside encroachments. Also, bridge class culverts require protection inside and outside the clear zone. If a bridge class culvert is outside the clear zone, consider increasing the offset of the metal beam guard fence to decrease the length of need. Maintain a 4-ft 0-in minimum distance away from the obstacle and provide a maximum slope of 1V:10H or flatter for the placement of the metal beam guard fence. If the bridge class culvert is outside the clear zone, Du equals the clear zone distance. If the bridge class culvert is inside the clear zone distance, Du equals the distance to the outside edge of the bridge class culvert.

Anchor: #i1005574

Design Equations

To determine the needed length of guard fence or barrier required for a given obstacle, design equations have been formulated for low volume (ADT 750 or less) and higher volume (ADT more than 750) conditions. A clear zone width of 16-ft and length of roadside travel of 200-ft are incorporated in the low volume design equation (for use on roadways when the present ADT volume is 750 or less). Also, if the clear zone required is less than 16-ft and the present ADT is 750 or less, use Equation A-1 for calculating the guard fence length of need.

Anchor: #i1036763Table A-2: Equations for Upstream and Downstream Length of Need

ADT < 750

Anchor: #JKPYUPJE

Equation A-2.

Anchor: #MEHMSHHX

Equation A-3.

ADT > 750

Anchor: #KENUMWFR

Equation A-4.

Anchor: #XOHFNKCR

Equation A-5.


Where:

    Anchor: #EPONUCWF

  • Lu = Length of guard fence needed (upstream of area of concern), ft

    • Anchor: #CIAFQBTN

  • Ld = Length of guard fence needed (downstream of area of concern), ft

    • Anchor: #FFCIWTET

  • Du = Distance from edge of travel lane to far side of area of concern or to outside edge of clear zone, whichever is least, ft (for upstream direction of traffic)

    • Anchor: #AULTNOII

  • Dd = Distance from edge of travel lane to far side of area of concern or to outside edge of clear zone, whichever is least, ft (for opposing direction of traffic)

    • Anchor: #GAKRJQCT

  • Gu = Guard fence offset from edge of travel lane adjacent to proposed guard fence, ft

    • Anchor: #SLPXXCBS

  • Gd = Guard fence offset from edge of opposing direction of travel lane (centerline), ft

For low volume conditions, if the clear zone width of 16-ft is met or exceeded, L=0.

For higher volumes, a clear zone width of 30-ft and length of roadside travel of 250-ft are incorporated into the design equation (for use on roadways when the present ADT volume is more than 750 or the recommended clear zone is greater than 16-ft).

For high volume conditions, if the clear zone width (30-ft) is met or exceeded, L=0.

Anchor: #i1005640

Using Design Equations to Determine Length of Guard Fence

Before determining length of guard fence, the designer should assemble the following pertinent data:

Once this design data has been assembled, the equation for length of guard fence can be used.

Where the prescribed length of the guard fence cannot be installed at a bridge end due to an intervening access point such as an intersecting roadway or driveway, the length of guard fence may be interrupted or reduced. This change in length is acceptable only in locations where the Department must meet the obligation to provide access and this access cannot be reasonably relocated. Alternative treatments in these situations include installing an appropriate radius rail, terminating the guard fence prior to the access location with an appropriate end treatment and continuing the guard fence beyond the access location if necessary, or using an alternate bridge end treatment. The selected treatment should consider potential sight line obstructions, crash history at the site, cost, and maintenance associated with the selected treatment. Reduced guard fence length to accommodate access points will not require a design exception or a design waiver.

Section 7, Example Problems provides example problems and solutions using the design equations. The guard fence lengths produced by the equations should be rounded up to an even length of guard fence. In circumstances where site conditions permit, the rounded-up length of need should terminate at the end of guard fence; any additional length of need component available from an end attenuator should be considered an additional buffer.

Previous page  Next page   Title page