Roadway Design Manual: Horizontal Alignment

Section 5: Horizontal Alignment

Overview

It is necessary to establish the proper relation between design speed and curvature when designing roadway alignment. The two basic elements of horizontal curves are:

Anchor: #OMQNICGR

Curve Radius and

Anchor: #FMWCUYPI

Superelevation Rate.

Anchor: #i1085879

General Considerations for Horizontal Alignment

There are a number of general considerations important for safe, smooth flowing, and aesthetically pleasing facilities. These practices as outlined below are particularly applicable to high-speed facilities.

Anchor: #FSYKGLYS

Flatter than minimum curvature for any particular design speed should be used where possible, while retaining the minimum guidelines for the most critical conditions;

Anchor: #CJLJYSKE

Alignment consistency should be sought. Sharp curves should not follow long tangents or a series of flat curves;

Anchor: #RHOWQUTF

Sharp curves should be avoided on long, high fills. It is difficult for drivers to perceive the extent of curvature and adjust their operation accordingly when the adjacent topography does not extend above the level of the roadway;

Anchor: #YEADLJWD

Compound curves (two adjacent curves in the same direction with different radii) should be used with caution and should be avoided on mainlanes where conditions permit the use of simple curves. Where compound curves are used, the ratio of the flatter radius to the sharper radius should not exceed 3:2 (i.e., R₁ should not exceed 1.5R₂). For intersections or other turning roadways (such as loops, connections, and ramps), this ratio may be increased to 2:1 (i.e., R₁ may be increased to 2R₂);

Anchor: #OWCOWYFM

Reverse curves (two adjacent curves in opposite directions) on high-speed facilities should include a tangent section of sufficient length to provide adequate superelevation transition between the curves;

Anchor: #POVWPCYC

Broken-back curves (two curves in the same direction with a short tangent between the curves) should be avoided if feasible. This configuration is unexpected by drivers, not pleasing in appearance, and more difficult for freight truck maneuverability; and

Anchor: #ASEBJQWT

Horizontal alignment and its associated design speed should be consistent with other design features and topography. Coordination with vertical alignment is discussed in Chapter 2, Section 6, Combination of Vertical and Horizontal Alignment.

Anchor: #BGBHGEGC

Curve Radius

The design of roadway curves should be based on an appropriate relationship between design speed and curvature as well as their joint relationships with superelevation rate and side friction. The minimum radii of curves are important control values in designing for safe operation. Design guidance for low-speed urban facilities (45-mph or less) is shown in Table 2-3. Design guidance for curvature of high-speed (50-mph or greater) or non-urban facilities is shown in Table 2-4 and Table 2-5 for maximum superelevation (e_max) rates equal to 6 percent and 8 percent respectively.

For high-speed design conditions, the maximum allowable deflection angle without a horizontal curve is 30 minutes. For low-speed design conditions, the maximum allowable deflection angle without a horizontal curve is 1 degree.

Anchor: #BGBIIDDD

Superelevation Rate

As a vehicle traverses a horizontal curve, it undergoes a centripetal acceleration that acts toward the center of the curve. Vehicle weight, roadway superelevation, and side friction between the tires and pavement surface sustain this acceleration. The equation that governs vehicle operation on a horizontal curve is:

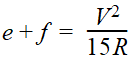
Where:

e = superelevation rate, ft/ft

f = side friction factor

V = vehicle speed, mph

R = curve radius, ft

There are practical upper limits to the rate of superelevation. The Department normally uses a maximum superelevation rate of 6 percent. However, a maximum rate of 8 percent may be used where higher superelevation rates or sharper curves are desired. The recommended maximum for facilities where there is a regular occurrence of very-slow moving vehicles, whose operation might be affected by high superelevation rates is 6 percent. Use of 8 percent should be coordinated with the District Design Engineer prior to implementation and documented in the project files.

Superelevation Rates on Low-Speed Urban Facilities

Although superelevation is advantageous for traffic operations, various factors often combine to make its use impractical in many urban areas. These factors include the following:

Anchor: #DSGPSHCA

Wide pavement areas;

Anchor: #WIQELDUX

Surface drainage considerations;

Anchor: #IUPMCXBW

Frequency of cross streets and driveways; and

Anchor: #CXSBTRJH

Need to meet the grade of adjacent property.

For these reasons, horizontal curves on low-speed urban facilities are frequently designed with normal crown. The centripetal acceleration, in this case, is counteracted solely with side friction. The term “normal crown” (NC) represents an equal downward pavement cross-slope, typically 2 percent, on each side of the axis of rotation.

Low-speed urban facilities should be designed using normal crown, such that superelevation is not necessary where practical. This is accomplished by using the negative e-values from Table 2-3. However, when superelevation is needed, a maximum superelevation rate of 4 percent should be used. This is accomplished by using the positive e-values from Table 2-3 to determine the superelevation rate for specific curvature and design speed conditions.

Table 2-3 shows the relationship of radius, superelevation rate, and design speed for low-speed urban facility design and should be used to evaluate existing conditions or the need for superelevation for proposed conditions on low-speed urban facilities. This table may also be used for design of detour alignments in constrained conditions.

Anchor: #AQNODTJD

Example:
Given a design speed of 35 mph and a 400 ft radius curve, Table 2-3 indicates an approximate superelevation rate of 2.4 percent should be used.

For a normal crown section, the negative e-value (the slope on the outside of the curve) will always be the controlling value for a given design speed.

Anchor: #XSWOITEH

Example:
Given a design speed of 45 mph and 1,050-ft radius curve, Table 2-3 indicates that a normal crown of 2 percent cross slope in each direction should be used.

Anchor: #i1321746Table 2-3: Minimum Radii and Superelevation Rates¹ for Low-Speed Urban Facilities
Design Speed
e (%)	15 mph R (ft)	20 mph R (ft)	25 mph R (ft)	30 mph R (ft)	35 mph R (ft)	40 mph R (ft)	45 mph R (ft)
-4.0²	54	116	219	375	583	889	1,227
-3.0²	52	111	208	353	544	821	1,125
-2.8²	51	110	206	349	537	808	1,107
-2.6²	51	109	204	345	530	796	1,089
-2.5^2,3	51	109	203	343	527	790	1,080
-2.4²	51	108	202	341	524	784	1,071
-2.2²	50	108	200	337	517	773	1,055
-2.0	50	107	198	333	510	762	1,039
-1.5^4,5	49	105	194	324	495	736	1,000
-1.0^4,5	48	103	189	316	480	711	964
-0.5^4,5	48	101	185	308	467	688	931
0^5,6	47	99	181	300	454	667	900
0.5⁵	46	97	177	293	441	646	871
1.0⁵	45	95	174	286	430	627	844
1.5⁵	45	94	170	279	419	610	818
2.0	44	92	167	273	408	593	794
2.2	44	91	165	270	404	586	785
2.4	44	91	164	268	400	580	776
2.6	43	90	163	265	396	573	767
2.8	43	89	161	263	393	567	758
3.0	43	89	160	261	389	561	750
3.2	43	88	159	259	385	556	742
3.4	42	88	158	256	382	550	734
3.6	42	87	157	254	378	544	726
3.8	42	87	155	252	375	539	718
4.0	42	86	154	250	371	533	711
Notes: Computed using Superelevation Distribution Method 2. See AASHTO's A Policy on Geometric Design of Highways and Streets for the different types of Superelevation Distribution Methods. Normal crown values beyond -2.0% should be used for surfaces such as gravel, crushed stone, and earth. Areas with paved surfaces that receive more frequent rainfall events with high intensities and greater depths than other areas may use 2.5% normal crown. For the purpose of evaluating existing conditions, normal crown values up to -1.5% may be used. Values ranging from -1.5% to +1.5% should only be used in special circumstances such as intersections. 0% is provided for information purposes only and should not be used for design.

Superelevation Rate on High-Speed or Non-Urban Facilities

Table 2-4 and 2-5 show superelevation rates (maximum 6 percent and 8 percent, respectively) for various design speeds and radii. These tables should be used for high-speed or non-urban facilities. For multi-lane facilities, particularly where wide medians are used, the radius applies to the inside edge of the innermost travel lane.

Anchor: #i1431298Table 2-4: Minimum Radii and Superelevation Rates¹for High-Speed or Non-Urban Facilities, e_max = 6%
Design Speed
e (%)	15 mph R (ft)	20 mph R (ft)	25 mph R (ft)	30 mph R (ft)	35 mph R (ft)	40 mph R (ft)	45 mph R (ft)	50 mph R (ft)	55 mph R (ft)	60 mph R (ft)	65 mph R (ft)	70 mph R (ft)	75 mph R (ft)	80 mph R (ft)
NC^3,4	868	1,580	2,290	3,130	4,100	5,230	6,480	7,870	9,410	11,100	12,600	14,100	15,700	17,400
RC^3,4	614	1,120	1,630	2,240	2,950	3,770	4,680	5,700	6,820	8,060	9,130	10,300	11,500	12,900
2.2	543	991	1,450	2,000	2,630	3,370	4,190	5,100	6,110	7,230	8,200	9,240	10,400	11,600
2.4	482	884	1,300	1,790	2,360	3,030	3,770	4,600	5,520	6,540	7,430	8,380	9,420	10,600
2.6	430	791	1,170	1,610	2,130	2,740	3,420	4,170	5,020	5,950	6,770	7,660	8,620	9,670
2.8	384	709	1,050	1,460	1,930	2,490	3,110	3,800	4,580	5,440	6,200	7,030	7,930	8,910
3.0	341	635	944	1,320	1,760	2,270	2,840	3,480	4,200	4,990	5,710	6,490	7,330	8,260
3.2	300	566	850	1,200	1,600	2,080	2,600	3,200	3,860	4,600	5,280	6,010	6,810	7,680
3.4	256	498	761	1,080	1,460	1,900	2,390	2,940	3,560	4,250	4,890	5,580	6,340	7,180
3.6	209	422	673	972	1,320	1,740	2,190	2,710	3,290	3,940	4,540	5,210	5,930	6,720
3.8	176	358	583	864	1,190	1,590	2,010	2,490	3,040	3,650	4,230	4,860	5,560	6,320
4.0	151	309	511	766	1,070	1,440	1,840	2,300	2,810	3,390	3,950	4,550	5,220	5,950
4.2	131	270	452	684	960	1,310	1,680	2,110	2,590	3,140	3,680	4,270	4,910	5,620
4.4	116	238	402	615	868	1,190	1,540	1,940	2,400	2,920	3,440	4,010	4,630	5,320
4.6	102	212	360	555	788	1,090	1,410	1,780	2,210	2,710	3,220	3,770	4,380	5,040
4.8	91	189	324	502	718	995	1,300	1,640	2,050	2,510	3,000	3,550	4,140	4,790
5.0	82	169	292	456	654	911	1,190	1,510	1,890	2,330	2,800	3,330	3,910	4,550
5.2	73	152	264	413	595	833	1,090	1,390	1,750	2,160	2,610	3,120	3,690	4,320
5.4	65	136	237	373	540	759	995	1,280	1,610	1,990	2,420	2,910	3,460	4,090
5.6	58	121	212	335	487	687	903	1,160	1,470	1,830	2,230	2,700	3,230	3,840
5.8	51	106	186	296	431	611	806	1,040	1,320	1,650	2,020	2,460	2,970	3,560
6.0	39	81	144	231	340	485	643	833	1,060	1,330	1,660	2,040	2,500	3,050
Notes: Computed using Superelevation Distribution Method 5. See AASHTO's A Policy on Geometric Design of Highways and Streets for the different types of Superelevation Distribution Methods. The term "NC" (normal crown) represents an equal downward cross-slope, typically 2%, on each side of the axis of rotation. The minimum curve radii for normal crown are suitable up to 3.0%. 3.0% normal crown should only be used when 3 or more lanes are sloped in the same direction. 1.5% or flatter normal crown should only be used for the design of special circumstance, such as table-topping intersections, or the evaluation of existing conditions. The term "RC" (reverse crown) represents a curve where the downward, or adverse, cross-slope should be removed by superelevating the entire roadway at the normal cross-slope rate. For curve radii falling between normal crown and reverse crown, rather than interpolation a superelevation rate equal to the normal crown should typically be used.

Anchor: #i1569750Table 2-5: Minimum Radii and Superelevation Rates¹for High-Speed or Non-Urban Facilities, e_max = 8%
Design Speed
e (%)	15 mph R(ft)	20 mph R(ft)	25 mph R(ft)	30 mph R(ft)	35 mph R(ft)	40 mph R(ft)	45 mph R(ft)	50 mph R(ft)	55 mph R(ft)	60 mph R(ft)	65 mph R(ft)	70 mph R(ft)	75 mph R(ft)	80 mph R(ft)
NC^2,4	932	1,640	2,370	3,240	4,260	5,410	6,710	8,150	9,720	11,500	12,900	14,500	16,100	17,800
RC^3,4	676	1,190	1,720	2,370	3,120	3,970	4,930	5,990	7,150	8,440	9,510	10,700	12,000	13,300
2.2	605	1,070	1,550	2,130	2,800	3,570	4,440	5,400	6,450	7,620	8,600	9,660	10,800	12,000
2.4	546	9,59	1,400	1,930	2,540	3,240	4,030	4,910	5,870	6,930	7,830	8,810	9,850	11,000
2.6	496	872	1,280	1,760	2,320	2,960	3,690	4,490	5,370	6,350	7,180	8,090	9,050	10,100
2.8	453	796	1,170	1,610	2,130	2,720	3,390	4,130	4,950	5,850	6,630	7,470	8,370	9,340
3.0	415	730	1,070	1,480	1,960	2,510	3,130	3,820	4,580	5,420	6,140	6,930	7,780	8,700
3.2	382	672	985	1,370	1,820	2,330	2,900	3,550	4,250	5,040	5,720	6,460	7,260	8,130
3.4	352	620	911	1,270	1,690	2,170	2,700	3,300	3,970	4,700	5,350	6,050	6,800	7,620
3.6	324	572	845	1,180	1,570	2,020	2,520	3,090	3,710	4,400	5,010	5,680	6,400	7,180
3.8	300	530	784	1,100	1,470	1,890	2,360	2,890	3,480	4,140	4,710	5,350	6,030	6,780
4.0	277	490	729	1,030	1,370	1,770	2,220	2,720	3,270	3,890	4,450	5,050	5,710	6,420
4.2	255	453	678	955	1,280	1,660	2,080	2,560	3,080	3,670	4,200	4,780	5,410	6,090
4.4	235	418	630	893	1,200	1,560	1,960	2,410	2,910	3,470	3,980	4,540	5,140	5,800
4.6	215	384	585	834	1,130	1,470	1,850	2,280	2,750	3,290	3,770	4,310	4,890	5,530
4.8	193	349	542	779	1,060	1,390	1,750	2,160	2,610	3,120	3,590	4,100	4,670	5,280
5.0	172	314	499	727	991	1,310	1,650	2,040	2,470	2,960	3,410	3,910	4,460	5,050
5.2	154	284	457	676	929	1,230	1,560	1,930	2,350	2,820	3,250	3,740	4,260	4,840
5.4	139	258	420	627	870	1,160	1,480	1,830	2,230	2,680	3,110	3,570	4,090	4,640
5.6	126	236	387	582	813	1,090	1,390	1,740	2,120	2,550	2,970	3,420	3,920	4,460
5.8	115	216	358	542	761	1,030	1,320	1,650	2,010	2,430	2,840	3,280	3,760	4,290
6.0	105	199	332	506	713	965	1,250	1,560	1,920	2,320	2,710	3,150	3,620	4,140
6.2	97	184	308	472	669	909	1,180	1,480	1,820	2,210	2,600	3,020	3,480	3,990
6.4	89	170	287	442	628	857	1,110	1,400	1,730	2,110	2,490	2,910	3,360	3,850
6.6	82	157	267	413	590	808	1,050	1,330	1,650	2,010	2,380	2,790	3,240	3,720
6.8	76	146	248	386	553	761	990	1,260	1,560	1,910	2,280	2,690	3,120	3,600
7.0	70	135	231	360	518	716	933	1,190	1,480	1,820	2,180	2,580	3,010	3,480
7.2	64	125	214	336	485	672	878	1,120	1,400	1,720	2,070	2,470	2,900	3,370
7.4	59	115	198	312	451	628	822	1,060	1,320	1,630	1,970	2,350	2,780	3,250
7.6	54	105	182	287	417	583	765	980	1,230	1,530	1,850	2,230	2,650	3,120
7.8	48	94	164	261	380	533	701	901	1,140	1,410	1,720	2,090	2,500	2,970
8.0	38	76	134	214	314	444	587	758	960	1,200	1,480	1,810	2,210	2,670
Notes: Computed using Superelevation Distribution Method 5. See AASHTO's A Policy on Geometric Design of Highways and Streets for the different types of Superelevation Distribution Methods. The term "NC" (normal crown) represents an equal downward cross-slope, typically 2%, on each side of the axis of rotation. The minimum curve radii for normal crown are suitable up to 3.0%. 3.0% normal crown should only be used when 3 or more lanes are sloped in the same direction. 1.5% or flatter normal crown should only be used for the design of special circumstance, such as table-topping intersections, or the evaluation of existing conditions. The term "RC" (reverse crown) represents a curve where the adverse, or negative, cross-slope should be removed by superelevating the entire roadway at the normal cross-slope rate. For curve radii falling between normal crown and reverse crown, a superelevation rate equal to the normal crown should typically be used.

Superelevation Transition Length

Superelevation transition is the general term denoting the change in cross slope from a normal crown section to the full superelevated section or vice versa. To meet the requirements of comfort and safety, the superelevation transition should occur over a length adequate for the usual travel speeds. Transition lengths should also account for potential future traveled way widening, including widening associated with the ultimate typical section in a schematic.

Desirable design values for length of superelevation transition are based on a given maximum relative gradient between profiles of the edge of traveled way and the axis of rotation. Table 2-6 shows recommended maximum relative gradient values. Transition length on this basis is directly proportional to the total superelevation, which is the product of the lane width and the change in cross slope.

Anchor: #i1548651Table 2-6: Maximum Relative Gradient (G) for Superelevation Transition
Design Speed (mph)	Maximum Relative Gradient¹ (%)	Equivalent Maximum Relative Slope (run:rise)
15	0.89	1:112
20	0.80	1:125
25	0.73	1:137
30	0.67	1:150
35	0.62	1:162
40	0.57	1:175
45	0.53	1:187
≥50	0.50	1:200
Note: Maximum relative gradient for profile between edge of traveled way and axis of rotation.

Desirable transition length, L_CT can be calculated using the following equation:

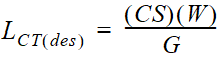
Where:

L_CT = Calculated desirable transition length, ft

CS = Change in cross slope of superelevated pavement, percent

W = distance between the axis of rotation and the edge of traveled way, ft

G = maximum relative gradient ( Table 2-6).

Example determinations of superelevation transition are shown in Figure 2-2.

Anchor: #SHEQIPOIgrtop

Figure 2-2. Determination of Length of Superelevation Transition.

As the number of lanes to be transitioned increases, the length of superelevation transition increases proportionately with the increased width. While strict adherence to the length (L_CT) calculation is desirable, the length for multi-lane facilities may become impractical for design purposes (e.g., drainage problems, avoiding bridges, accommodating merge/diverge condition). A minimum length (L_CT), can be calculated using adjustment factors as shown in Table 2-7, such that the transition length formula becomes:

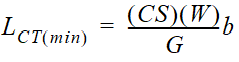
where “b” is defined in Table 2-7. In the case of one lane being rotated, “b” is 1.0, such that L_{CT (min)} = L_{CT (des)}

Anchor: #i1357549Table 2-7: Multilane Adjustment Factor¹
Number of Lanes Rotated (n)	Adjustment Factor (b)
1.5	0.83
2	0.75
2.5	0.70
3	0.67
3.5	0.64
Note: These adjustment factors are directly applicable to undivided facilities. For divided facilities where the axis of rotation is not the edge of traveled way, see AASHTO’s A Policy on Geometric Design of Highways and Streets.

Anchor: #EFCGXLVU

Superelevation Transition Placement

The transition with respect to the termini of a simple (circular) curve should be placed to minimize lateral acceleration and the vehicle's lateral motion. The recommended allocation of superelevation transition on the tangent, preceding or following a curve, is provided on Table 2-8. For superelevation on bridge structures, it is preferred to begin/end superelevation at the bridge bent line. When spiral curves are present on an existing facility and alignment modifications aren't practical, refer to AASHTO’s A Policy on Geometric Design of Highways and Streets for transition distribution.

Anchor: #i1550164Table 2-8: Portion of Superelevation Transition Located on the Tangent¹
Design Speed (mph)	No. of Lanes Rotated
	1.0	1.5	2.0 - 2.5	3.0 - 3.5
15 - 45	0.80	0.85	0.90	0.90
50 - 80	0.70	0.75	0.80	0.85
Note: These values are recommendations based on prevailing research. A value between 0.7 and 0.9 for all speeds and rotated widths is considered acceptable. Refer to AASHTO’s A Policy on Geometric Design of Highways and Streets for additional information.

Care must be exercised in designing the length and location of the superelevation transition. Pavement surfaces should be modeled to ensure proper drainage, especially near the high or low portions of Type I or III vertical curves (see Figure 2-5 for curve types). A plot of roadway contours may assist with the verification of grades and identification of drainage problems in areas of superelevation transition. Desirably, a minimum profile grade line (PGL) of 0.5 percent and minimum edge-of-pavement (EOP) profile grade of 0.2 percent (0.5 percent for curbed roadways) should be maintained throughout the transition section. At a minimum, either criterion should be met.

Whenever reverse curves are closely spaced and superelevation transition lengths overlap, transition lengths (L_CT) should be adjusted to ensure that roadway cross slopes are in the proper direction for each horizontal curve. For proposed construction of new facilities, the tangent section between reverse curves should be of sufficient length such that minimum transition lengths for each transition do not overlap.

Anchor: #YWUECXWM

Superelevation Transition Type

Linear or reverse parabolic transitions may be used for attaining superelevation. Where appearance is a factor (e.g., curbed sections and retaining walls) use of reverse parabolic is recommended. This produces an outer edge profile that is smooth, undistorted, and pleasing in appearance. However, for bridges, linear transitions are generally preferred for constructability, ride quality, and lower cost. Notate the transition type in the plans to ensure the transition is properly constructed.

Figure 2-2 shows reverse parabolic and linear transitions over the full length of the transition. Refer to AASHTO’s A Policy on Geometric Design of Highways and Streets for alternative methods for developing smooth-edge profiles over the length of the transition.

Anchor: #i1086199

Sight Distance on Horizontal Curves

Where an object off the pavement restricts sight distance, such as a bridge pier, bridge railing, median barrier, retaining wall, building, cut slope or natural growth, the minimum radius of curvature is determined by the stopping sight distance.

The following equation applies only to circular curves longer than the stopping sight distance (S<L) for the pertinent design speed. For example, with a 50-mph design speed and a curve with a 1,150-ft radius, a clear sight area with a horizontal sight line offset (HSO) of approximately 20-ft is needed for stopping sight distance.

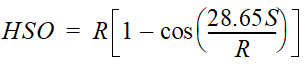
Where:

HSO = horizontal sight line offset, ft

S = stopping sight distance (Table 2-1), ft

R = radius at centerline of inner most travel lane, ft

This method for calculating HSO is only exact when both the vehicle and sight obstruction are located within the horizontal curve. When the vehicle or sight obstruction are located outside of the horizontal curve (i.e. S>L) this method will result in an HSO slightly larger than required. In many instances the resulting additional clearance will not be significant. In some cases, the design should be checked either by using graphical procedures or computational methods to verify HSO. NHCRP 910 provides computational methods for verifying HSO.

In cases where complex geometries or discontinuous objects cause sight obstructions, graphical methods may be useful in determining available sight distance and associated offset requirements. Graphical methods may also be used when the circular curve is shorter than the stopping sight distance.

To check horizontal sight distance on the inside of a curve graphically, sight lines equal to the required sight distance on horizontal curves should be reviewed to ensure that obstructions such as buildings, hedges, barrier railing, and high ground do not restrict the sight distance required in either direction. Figure 2-3 illustrates a graphical approach to determining horizontal sight distance in a curve.

Anchor: #FSJVSJGTgrtop

Figure 2-3. Diagram Illustrating Components for Determining Horizontal Sight Distance. Source: AASHTO's A Policy on Geometric Design of Highways and Streets

Where sufficient stopping sight distance is not available because a railing, longitudinal barrier or other features constitutes a sight obstruction, alternative designs should be considered. Potential alternatives include: (1) increasing the offset to the obstruction or (2) increasing the radius. However, the alternative should not incorporate a shoulder width on the inside of the curve in excess of 12-ft because of the concern that drivers will use wider shoulders as a passing or travel lane.