Anchor: #CHDHIHHI

Section 4: Sight Distance

Anchor: #i1085655

Overview

This section provides descriptions and information on sight distance, one of several principal elements of design that are common to all types of highways and streets. Of utmost importance in highway design is the arrangement of geometric elements so that there is adequate sight distance for safe and efficient traffic operation assuming adequate light, clear atmospheric conditions, and drivers' visual acuity. For design, the following five types of sight distance are considered:

Anchor: #CHDCDCCH

Stopping Sight Distance

Sight distance is the length of roadway ahead that is visible to the driver. The available sight distance on a roadway should be sufficiently long enough to enable a vehicle traveling at or near the design speed to stop before reaching a stationary object in its path.

Stopping sight distance is the sum of two distances:

    Anchor: #HLQOHWOF
  • Brake reaction distance – the distance traversed by the vehicle from the instant the driver sights an object necessitating a stop to the instant the brakes are applied.
    • Anchor: #JMQLUQRY
  • Braking distance – the distance needed to stop the vehicle from the instant brake application begins on level terrain.

Approximately 90% of all drivers decelerate at rates greater than 11.2 ft/s2. Such decelerations allow the driver to maintain steering control during the braking maneuver on wet surfaces. Therefore, 11.2 ft/s2 is recommended as the deceleration threshold for determining stopping sight distance.

In computing and measuring stopping sight distance, the height of the driver’s eye is estimated to be 3.5-ft and the height of the object to be seen by the driver is 2.0-ft, equivalent to the taillight height of passenger car.

The calculated and design stopping sight distances are shown in Table 2-1.

The values given in Table 2-1 represent stopping sight distance on level terrain. As a general rule, the sight distance available on downgrades is larger than on upgrades, therefore, corrections for grade are usually unnecessary. An example where correction for grade might be applicable for stopping sight distance would be a divided roadway with independent design profiles in extreme rolling or mountainous terrain. AASHTO’s A Policy on Geometric Design for Highways and Streets, provides additional information and suggested values for grade corrections in these rare circumstances.

Anchor: #i1319617Table 2-1: Stopping Sight Distance on Level Roadways

Design Speed

(mph)

Brake Reaction Distance1

(ft)

Braking Distance

(ft)

Stopping Sight Distance

Calculated

(ft)

Design

(ft)

15

55.1

21.6

76.7

80

20

73.5

38.4

111.9

115

25

91.9

60.0

151.9

155

30

110.3

86.4

196.7

200

35

128.6

117.6

246.2

250

40

147.0

153.6

300.6

305

45

165.4

194.4

359.8

360

50

183.8

240.0

423.8

425

55

202.1

290.3

492.4

495

60

220.5

345.5

566.0

570

65

238.9

405.5

644.4

645

70

257.3

470.3

727.6

730

75

275.6

539.9

815.5

820

80

294.0

614.3

908.3

910

Note:

  1. Brake reaction distance predicated on a time of 2.5-s; deceleration rate 11.2-ft/s²


Anchor: #CHDGIJJG

Decision Sight Distance

Decision sight distance is the distance required for a driver to detect an unexpected or otherwise difficult-to-perceive information source, recognize the source, select an appropriate speed and path, and initiate and complete the required maneuver safely and efficiently. Because decision sight distance gives drivers additional margin for error and affords them sufficient length to maneuver their vehicles at the same or reduced speed rather than to just stop, its values are substantially greater than stopping sight distance. Table 2-2 shows recommended decision sight distance values for various avoidance maneuvers.

Anchor: #i1318657Table 2-2: Recommended Decision Sight Distance Values

Avoidance Maneuver Decision Sight Distance (ft)

Design Speed (mph)

A1

B2

C3

D4

E5

30

220

490

450

535

620

35

275

590

525

625

720

40

330

690

600

715

825

45

395

800

675

800

930

50

465

910

750

890

1030

55

535

1030

865

980

1135

60

610

1150

990

1125

1280

65

695

1275

1050

1220

1365

70

780

1410

1105

1275

1445

75

875

1545

1180

1365

1545

80

970

1685

1260

1455

1650

Notes:

  1. Avoidance Maneuver A: Stop on rural road; t = 3.0s
  2. Avoidance Maneuver B: Stop on urban road; t = 9.1s
  3. Avoidance Maneuver C: Speed/path/direction change on rural road; t varies between 10.2 and 11.2s
  4. Avoidance Maneuver D: Speed/path/direction change on suburban road; t varies between 12.1 and 12.9s
  5. Avoidance Maneuver E: Speed/path/direction change on urban road; t varies between 14.0 and 14.5s
  6. t = time in seconds


Examples of situations in which decision sight distance is preferred include the following:

    Anchor: #MABVBBAU
  • Interchange and intersection locations where unusual or unexpected maneuvers are required (such as exit ramp gore areas and left-side exits)
    • Anchor: #OIFXXTTE
  • Changes in cross-section such as lane drops
    • Anchor: #DMKXBIPJ
  • Areas of concentrated demand where “visual noise” is present with competing sources of visual information, such as roadway elements, traffic elements, traffic control devices, and advertising signs

Locations along the roadway where a driver has stopping sight distance but not the extra response time provided by decision sight distance is identified as a “reduced decision zone”. Avoid placement of intersections within a reduced decision zone by relocating the intersection or by changing the grades to reduce the length of the reduced decision zone.

Anchor: #CHDBBGGG

Passing Sight Distance

Passing sight distance is applicable only in the design of two-lane roadways (including two-way frontage roads) and therefore is presented in Chapter 3 Section 4, Two Lane Rural Highways, and Chapter 4 Section 6, Super 2 Highways.

Anchor: #BGBFDJGG

Intersection Sight Distance

The operator of a vehicle approaching an intersection should have an unobstructed view of the entire intersection and an adequate view of the intersecting highway to permit control of the vehicle to avoid a collision. This visibility is referred to as intersection sight distance. When designing an intersection, the following factors should be taken into consideration:

    Anchor: #HAENPROX
  • Adequate sight distance should be provided along both highway approaches and across corners;
    • Anchor: #JDTVEMXW
  • Gradients of intersecting highways should be as flat as practical on sections that are to be used for storage of stopped vehicles;
    • Anchor: #WEDTMNAR
  • Combination of vertical and horizontal curvature should allow adequate sight distance of the intersection;
    • Anchor: #RRBEVUWG
  • Traffic lanes and marked pedestrian crosswalks should be clearly visible at all times;
    • Anchor: #JTAAAJUW
  • Lane markings and signs should be clearly visible and understandable from a desired distance;
    • Anchor: #BVJTXUGB
  • Intersections should eliminate, relocate or modify conflict points to the extent allowable in order to improve safety; and
    • Anchor: #PNYOKDIJ
  • Intersections should be evaluated for the effects of barriers, rails, and retaining walls on sight distance.

For selecting intersection sight distance, refer to AASHTO’s A Policy on Geometric Design for Highways and Streets. Sight distance criteria are provided for the following types of intersection controls:

Anchor: #i1701009

Sight Distance at Under-Crossings

Sight distance through a grade crossing should be at least the minimum stopping sight distance, or longer. Line of sight may be obstructed by an overpass structure and can limit the sight distance for the operator. Where practical, provide the minimum length of sag vertical curve at grade separated structures. Where economically feasible, for two-lane roadways, the passing sight distance should be maintained (see Chapter 3, Section 4 – Passing Sight Distances).

When the minimum sag vertical curve length based on headlight sight distance, as described in Section 6, is achieved for vertical clearances of 14’ or higher, then Figure 2.1 and subsequent equations are not applicable. However, if any of the following conditions occur, then the under-crossing curve length as shown in Figure 2-1 and subsequent equations should be verified based on the applicable sight distance (SSD):

    Anchor: #RTVMQOCR
  • The minimum sag vertical curve length based on SSD for headlights is not met
    • Anchor: #TBNOGAOM
  • The comfort control (lighting) criteria is used to establish the sag vertical curve length
    • Anchor: #HGLOUWQP
  • The vertical clearance is less than 14 feet

Sight Distance at Under-crossings. Source: AASHTO’s A Policy on Geometric Design of Highways and Streets (click in image to see full-size image) Anchor: #JFHGVJMHgrtop

Figure 2-1. Sight Distance at Under-crossings. Source: AASHTO’s A Policy on Geometric Design of Highways and Streets

The general equations for sag vertical curve length at under-crossings are:

Case 1 – Sight distance greater than length of vertical Curve (S>L):

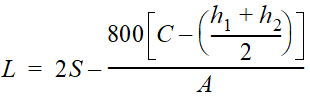
Where:

Case 2 – Sight distance less than length of vertical curve (S<L)

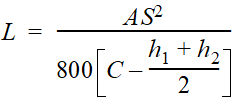
Using an eye height of 8.0 ft for a truck driver and an object height of 2.0 ft for the taillights of a vehicle, the following equations can be derived:

Case 1 – Sight distance greater than length of vertical curve (S>L):

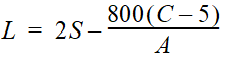
Case 2 – Sight distance less than length of vertical curve (S<L):

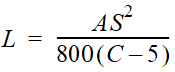
Where:
Previous page  Next page   Title page