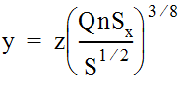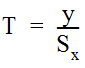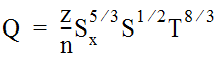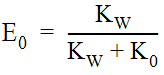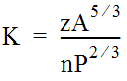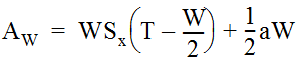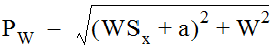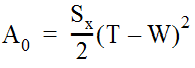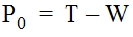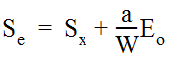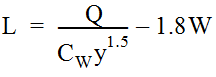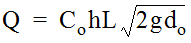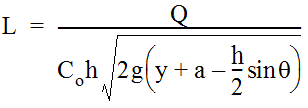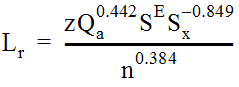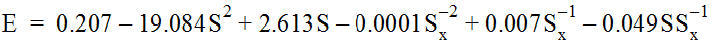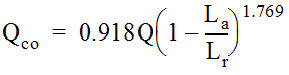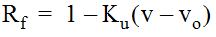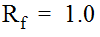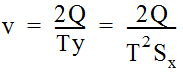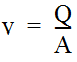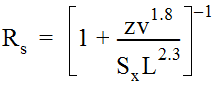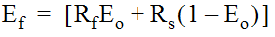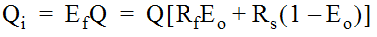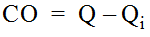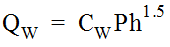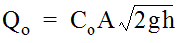Anchor: #i1017022

Section 6: Gutter and Inlet Equations

Anchor: #i1017027

Gutter Flow

The ponded width is a geometric function of the depth of the water (y) in the curb and gutter section. The spread is usually referred to as ponded width (T), as shown in Figure 10-10.

Gutter Flow Cross Section Definition of Terms (click in image to see full-size image) Anchor: #PPUISUBXgrtop

Figure 10-13. Gutter Flow Cross Section Definition of Terms

Using Manning's Equation for Depth of Flow as a basis, the depth of flow in a curb and gutter section with a longitudinal slope (S) is taken as the uniform (normal) depth of flow. (See Chapter 6 for more information.) For Equation 10-1, the portion of wetted perimeter represented by the vertical (or near-vertical) face of the curb is ignored. This justifiable expedient does not appreciably alter the resulting estimate of depth of flow in the curb and gutter section.

Anchor: #HWCMUNBD

Equation 10-1.

where:

The table below presents suggested Manning's “n” values for various pavement surfaces. Department recommendation for design is the use of the rough texture values.

Anchor: #i1124023Table 10-1 Manning’s n-Values for Street and Pavement Gutters

Type of gutter or pavement

n

asphalt pavement:

Smooth texture

Rough texture

0.013

0.016

Concrete gutter with asphalt pavement:

Smooth texture

Rough texture

0.013

0.015

Concrete pavement:

Float finish

Broom finish

0.014

0.016


Refer to Figure 10-10, and translate the depth of flow to a ponded width on the basis of similar triangles using Equation 10-2. Equation 10-2 can also be used to determine the ponded width in a sag configuration, where “y” is the depth of standing water or head on the inlet.

Anchor: #SBLAFBYV

Equation 10-2.

where:

Equations 10-1 and 10-2 are combined to compute the gutter capacity.

Anchor: #XWBGXEEF

Equation 10-3.

where:

Rearranging Equation 10-3 gives a solution for the ponded width, “T”.

Anchor: #OTGCLNHF

Equation 10-4.

where:

Equations 10-3 and 10-4 apply to roadway sections having constant cross slope and a vertical curb. The FHWA publication “Urban Drainage Design Manual" ( HEC-22) should be consulted for parabolic and other shape roadway sections.

Anchor: #i1017082

Ponding on Continuous Grades

Excessive ponding on continuous grades can be avoided by proper placement of storm drain inlets. The gutter ponding at a specific location may be determined on a continuous grade using the following steps:

    Anchor: #PAMJSDQC
  1. Select a location of a proposed inlet. Start on the high point and work towards the low point. Take into consideration the discussion in Inlet Locations.
    2. Anchor: #IMIEDJDG
  2. Calculate the total discharge in the gutter based on the drainage area to the selected location. See Determination of Runoff for methods to calculate discharge.
    3. Anchor: #XYRNJDAF
  3. Determine the longitudinal slope, transverse slope and Manning's roughness coefficient of the gutter.
    4. Anchor: #SIAFLODB
  4. Compute the ponded depth and width. For a constant transverse slope, compute the ponded depth using Equation 10-1 and the ponded width using Equation 10-2. For parabolic gutters or sections with more than one transverse slope, refer to the FHWA publication “Urban Drainage Design Manual" ( HEC-22).
Anchor: #i1017117

Ponding at Approaches to Sag Locations

Because a sag location has a different amount of flow approaching from both directions, the gutter to each side of the inlet has a different ponded width and depth. At sag locations, the hydraulic designer must consider sag inlet capacity and allowable ponding using the following steps:

    Anchor: #SJCLOXHD
  1. Estimate the apportionment of runoff to the left and right approaches. Next compute the discharge to the sag location based on the entire drainage area. Then determine the approximate fraction of area contributing to each side of the sag location. Multiply the total discharge by each fraction to determine the discharge to each side.
    2. Anchor: #YUCXGTDJ
  2. Determine the longitudinal slope of each gutter approach. For sawtooth profiles, the slopes will be the profile grades of the left and right approaches. However, if the sag is in a vertical curve, the slope at the sag will be zero, which would mean no gutter capacity. In reality there is a three-dimensional flow pattern resulting from the drawdown effect of the inlet. As an approximation, assume a longitudinal slope of one half of the tangent grade.
    3. Anchor: #ITVDUGER
  3. For each side of the sag, calculate the ponded depth and width using the appropriate flow apportionment, longitudinal slope, and Equation 10-1. Compute the ponded width using Equation 10-2.
Anchor: #i1017155

Ponded Width Confirmation

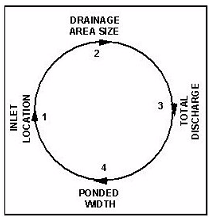
Figure 10-11 shows the interdependence of inlet location, drainage area, discharge, and ponded width. A tentative inlet location is selected, drainage area determined for that location, discharge established, and ponded width calculated. Once the ponded width has been calculated for a tentative location, it must be compared to the allowable ponded width and depth.

If the ponded width is exceeded, the design must be adjusted by relocating the inlet to a point upstream in the curb and gutter section which will reduce the watershed area, the peak discharge, and thus the ponded width. This process is repeated until the ponded width is at or below the allowable ponded width.

Relation of Inlet Location to Design Discharge (click in image to see full-size image) Anchor: #KNXOWNMKgrtop

Figure 10-14. Relation of Inlet Location to Design Discharge

If the calculated ponded width is less than or equal to the allowable ponded width, the hydraulic designer must decide if the design is efficient. If all or most of the allowable ponded width is used, the location is probably efficient. If only a small portion of the allowable ponded width is used, a more efficient location may be possible. In extensive storm drain systems, a design objective should be to minimize the number of inlets without violating allowable ponded widths anywhere in the system. (See Ponding for guidelines on allowable ponding.)

Anchor: #i1017165

Carryover Design Approach

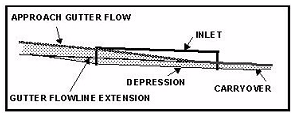
An on-grade inlet may be much more efficient if it intercepts only a portion of the total flow in the gutter instead of all of the flow in the gutter. The gutter flow not intercepted is called bypass flow or carryover. This design approach can only be used for on-grade configurations and is recommended where interception of the total flow is not necessary.

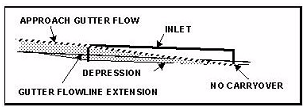
Figure 10-12 illustrates (in profile) approximately what happens when the inlet is designed to intercept all of the approaching flow. Note the large area of inlet opening that is not utilized efficiently.

Figure 10-13 illustrates (in profile) approximately what happens when the inlet is designed for bypass flow. Note that the inlet opening is used much more efficiently than the inlet illustrated in Figure 10-12.

Inlet Designed with No Carryover (click in image to see full-size image) Anchor: #LJILJSPTgrtop

Figure 10-15. Inlet Designed with No Carryover

Inlet Designed with Carryover (click in image to see full-size image) Anchor: #MTIAVORPgrtop

Figure 10-16. Inlet Designed with Carryover

Bypass flow is normally captured at some other location. The gutter between the two points must accommodate the additional flow. Bypass flow is not recommended upstream of intersections and driveways, at superelevation transitions where the cross slope begins to reverse, or below entrance/exit ramps. Bypass flow at these locations would be crossed by vehicular traffic and may pose a traffic hazard. Bypass flow is also not recommended to be allowed to flow where there is no outfall or designated capture point.

Anchor: #i1017364

Curb Inlets On-Grade

The design of on-grade curb opening inlets involves determination of length required for total flow interception, subjective decision about actual length to be provided, and determination of any resulting carryover rate.

The following procedure is used to design curb inlets on-grade:

    Anchor: #FPFOMNHE
  1. Compute depth of flow and ponded width (T) in the gutter section at the inlet.
    2. Anchor: #KKJYFPBQ
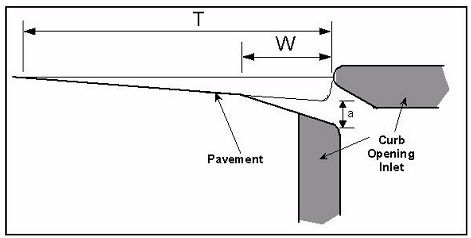
  2. Determine the ratio of the width of flow in the depressed section (W) to the width of total gutter flow (T) using Equation 10-5. Figure 10-14 shows the gutter cross section at an inlet.

    Anchor: #PEKXXNCH

    Equation 10-5.

    where:

    Gutter Cross-Section Diagram (click in image to see full-size image) Anchor: #AXJSLFQYgrtop

    Figure 10-17. Gutter Cross-Section Diagram

    Use Equation 10-6 to calculate conveyance, KW and K0.

    Anchor: #RDEOVFBQ

    Equation 10-6.

    where:

    Use Equation 10-7 to calculate the area of cross section in the depressed gutter section.

    Anchor: #EUIXQMGO

    Equation 10-7.

    where:

    Use Equation 10-8 to calculate the wetted perimeter in the depressed gutter section.

    Anchor: #MRLWSWDB

    Equation 10-8.

    where:

    Use Equation 10-9 to calculate the area of cross section of the gutter section beyond the depression.

    Anchor: #FOHMLYYT

    Equation 10-9.

    where:

    Use Equation 10-10 to calculate the wetted perimeter of the gutter section beyond the depression.

    Anchor: #BBVWYPYT

    Equation 10-10.

    where:

    3. Anchor: #KQAUBOKM
  3. Use Equation 10-11 to determine the equivalent cross slope (Se) for a depressed curb opening inlet.

    Anchor: #IGSBKWYE

    Equation 10-11.

    where:

    4. Anchor: #OMJIROFU
  4. Calculate the length of curb inlet required for total interception using Equation 10-12.

    Anchor: #TIUGQARR

    Equation 10-12.

    where:

    If no bypass flow is allowed, the inlet length is assigned a nominal dimension of at least Lr, which should be an available (nominal) standard curb opening length. The exact value of Lr should not be used if doing so requires special details, special drawings, structural design, and costly construction.

    If bypass flow is allowed, the inlet length is rounded down to the next available standard (nominal) curb opening length.

    5. Anchor: #DCTVUYRW
  5. Determine bypass flow. In bypass flow computations, efficiency of flow interception varies with the ratio of actual length of curb opening inlet supplied (La) to required length (Lr) and with the depression to depth of flow ratio. Use Equation 10-13 to calculate bypass flow.

    Anchor: #SNBADLAY

    Equation 10-13.

    where:

    Bypass flows usually should not exceed about 0.5 cfs (0.03 m3/s). Greater rates can be troublesome and cause a significant departure from the principles of the Rational Method application. In all cases, the bypass flow must be accommodated at some other specified point in the storm drain system.

    6. Anchor: #FWDBDLIT
  6. Calculate the intercepted flow as the original discharge in the approach curb and gutter minus the amount of bypass flow.
Anchor: #i1128377

Curb Inlets in Sag Configuration

The capacity of a curb inlet in a sag depends on the water depth at the curb opening and the height of the curb opening. The inlet operates as a weir to depths equal to the curb opening height and as an orifice at depths greater than 1.4 times the opening height. At depths between 1.0 and 1.4 times the opening height, flow is in a transition stage and the capacity should be based on the lesser of the computed weir and orifice capacity. Generally, for Department design, this ratio should be less than 1.4 such that the inlet operates as a weir.

    Anchor: #BMUDBINK
  1. If the depth of flow in the gutter (y) is less than or equal to 1.4 times the inlet opening height (h), ( 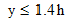), determine the length of inlet required considering weir control. Otherwise, skip this step. Calculate the capacity of the inlet when operating under weir conditions with Equation 10-14.

    Anchor: #ILRKWDJQ

    Equation 10-14.

    Equation 10-14 is rearranged to produce the following relation for curb inlet length required.

    Anchor: #SDRPNGHA

    Equation 10-15.

    where:
    2. Anchor: #CLKJPWKE
  2. If the depth of flow in the gutter is greater than the inlet opening height (y > h), determine the length of inlet required considering orifice control. The equation for interception capacity of a curb opening operating as an orifice follows:

    Anchor: #PFQRKYDE

    Equation 10-16.

    where:

    For curb inlets with an inclined throat such as Type C inlet, the effective head, do, is at the centroid of the orifice. This changes Equation 10-16 to:

    Anchor: #LFXHMJPR

    Equation 10-17.

    where: Q = total flow reaching inlet (cfs or m3/s) Co = orifice coefficient = 0.67 h = depth of opening (ft. or m)(this depth will vary slightly with the inlet detail used) L = length of curb opening inlet (ft. or m) g = acceleration due to gravity = 32.2 ft/s2 or 9.81 m/s2 y = depth of water in the curb and gutter cross section (ft. or m) a = gutter depression depth (ft.). Rearranging Equation 10-17 allows a direct solution for required length.

    3. Anchor: #GUQHYXIF

  3. Anchor: #VUVQDDEI

    Equation 10-18.

    4. Anchor: #CAHEGUDB
  4. If both steps 1 and 2 were performed (i.e., 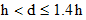), choose the larger of the two computed lengths as being the required length.
    5. Anchor: #DYJPSIWS
  5. Select a standard inlet length that is greater than the required length.
Anchor: #EOSFQXWX

Slotted Drain Inlet Design

The following procedure may be used for on-grade slotted drain inlets:

    Anchor: #BLTVCREQ
  1. Determine the length of slotted drain inlet required for interception of all of the water in the curb and gutter calculated by Equation 10-19.

    Anchor: #YQTMVSQP

    Equation 10-19.

    Equation 10-19 is limited to the following ranges of variables:

    Anchor: #BDABLYFN

    Equation 10-20.

    Because the equations are empirical, extrapolation is not recommended.

    2. Anchor: #QXLUXYAD
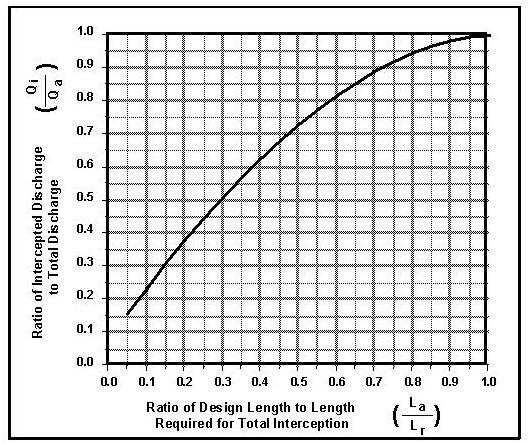
  2. Select the desired design slotted drain length (La) based on standard inlet sizes. If La < Lr the interception capacity may be estimated using Figure 10-15, multiplying the resulting discharge ratios by the total discharge. Alternatively, the carryover for a slotted drain inlet length may be directly computed using Equation 10-21.

    Anchor: #GMYKSPWE

    Equation 10-21.

    (click in image to see full-size image) Anchor: #SPYKLYUKgrtop

    Figure 10-18.

    As a rule of thumb, the hydraulic designer can optimize slotted drain inlet economy by providing actual lengths (La) to required lengths (Lr) in an approximate ratio of about 0.65. This implies a usual design with carryover for on-grade slotted drain inlets.

Anchor: #VNPKSFGR

Trench Drain Inlet Design

The following procedure may be used for trench drain design:

    Anchor: #MTLHKCYG
  1. Determine the location of trench drain to intercept the runoff.
    2. Anchor: #CVSSKMLE
  2. If on a slope, calculate the runoff to be captured by the trench drain in terms of cfs per foot of slope width. The maximum intercept rate, calculated from the weir equation, is 1.4 cfs per foot of length per side of trench drain for the Department specified grate. Flows exceeding 1.4 cfs per foot of length per side will require a different method of interception or multiple rows of trench drain inlets.
    3. Anchor: #CGSWNARM
  3. Select the outfall location for the trench drain. The maximum length of the trench drain is measured from the outfall location because the depth of the outfall, whether natural ground or a storm drain pipe, will determine the maximum depth of the trench drain. Trench drain is normally available with either a fixed invert slope or neutral invert without slope.
    4. Anchor: #NNFOWGSJ
  4. Look at the manufacturer's data to select the segments needed by part number.
    5. Anchor: #MLSRBHWP
  5. Select the trench drain outlet, horizontal or vertical, and size.
    6. Anchor: #DEJUAVOG
  6. Calculate the allowable flow in the drain outlet using the orifice equation.
    7. Anchor: #WGGSSFFS
  7. Select the method of connection to the storm drain pipe.
Anchor: #LNSBQESP

Grate Inlets On-Grade

The capacity of a grate inlet on-grade depends on its geometry and cross slope, longitudinal slope, total gutter flow, depth of flow, and pavement roughness.

The depth of water next to the curb is the major factor affecting the interception capacity of grate inlets. At low velocities, all of the water flowing in the section of gutter occupied by the grate, called frontal flow, and a small portion of the flow along the length of the grate, termed side flow, is intercepted. On steep slopes, only a portion of the frontal flow is intercepted if the velocity is high or the grate is short because a portion tends to splash over the end of the grate (“splash-over”). For grates less than 2 ft. (0.6 m) long, intercepted flow is small.

Refer to HEC-12 or HEC-22 for inlet efficiency data for various sizes and designs of grates. Additionally, safety for small wheeled vehicles (bicycles, wheelchairs, etc.) must be considered in grate selection.

Anchor: #GIENJDLU

Design Procedure for Grate Inlets On-Grade

Use the following procedure for grate inlets on-grade:

    Anchor: #PYUKFBFA
  1. Compute the ponded width of flow (T). Use the outline provided in Gutter Ponding on Continuous Grades.
    2. Anchor: #VIJGCHFP
  2. Choose a grate type and size.
    3. Anchor: #AMKMFLWC
  3. Find the ratio of frontal flow to total gutter flow (Eo) for a straight cross-slope using Equation 10-5. No depression is applied to a grate on-grade inlet.
    4. Anchor: #IFPBISPV
  4. Find the ratio of frontal flow intercepted to total frontal flow, Rf, using Equations 10-22, 10-23, and 10-24.

    If v > vo, use Equation 10-22.

    Anchor: #FQQNGSPV

    Equation 10-22.

    If v < vo, use Equation 10-23.

    Anchor: #VWMXSSRO

    Equation 10-23.

    where:

    For triangular sections, calculate the approach velocity of flow in gutter (v) using Equation 10-24.

    Anchor: #FWTMBBXP

    Equation 10-24.

    Otherwise, compute the section flow area of flow (A) and calculate the velocity using Equation 10-25:

    Anchor: #EPNKVUVU

    Equation 10-25.

    where:

      Anchor: #IOFDEGMK
    • vo = splash-over velocity (ft./s or m/s)
      • Anchor: #MVINKESP
    • L = length of grate (ft. or m)

      • Calculate the minimum velocity (vo) that will cause splash over the grate using the appropriate equation in Table 10-2 below.

    Anchor: #i1132824Table 10-2. Splash-Over Velocity Calculation Equations (English)

    Grate Configuration

    Typical Bar Spacing (in.)

    Splash-over Velocity Equation

    Parallel Bars

    2

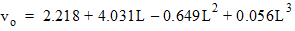
    Parallel Bars

    1.2

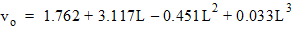
    Parallel bars w/ transverse rods

    2 parallel/4 transverse

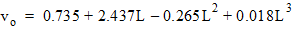
    Reticuline

    n/a



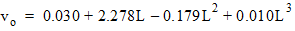    5. Anchor: #MPBFHRTJ
  5. Find the ratio of side flow intercepted to total side flow, Rs.

    Anchor: #VHXUWSNT

    Equation 10-26.

    where:

    6. Anchor: #OEEXADYG
  6. Determine the efficiency of grate, Ef. Use Equation 10-27.

    Anchor: #FIXTFVMU

    Equation 10-27.

    7. Anchor: #FQAONNKW
  7. Calculate the interception capacity of the grate, Qi. Use Equation 10-28. If the interception capacity is greater than the design discharge, skip step 8.

    Anchor: #JFYIMYUW

    Equation 10-28.

    8. Anchor: #SFJLGGJA
  8. Determine the bypass flow (CO) using Equation 10-29.

    Anchor: #HTQHJEEI

    Equation 10-29.

    9. Anchor: #RLASMDTC
  9. Depending on the bypass flow, select a larger or smaller inlet as needed. If the bypass flow is excessive, select a larger configuration of inlet and return to step 3. If the interception capacity far exceeds the design discharge, consider using a smaller inlet and return to step 3.
Anchor: #MQGUKBSH

Design Procedure for Grate Inlets in Sag Configurations

A grate inlet in sag configuration operates in weir flow at low ponded depths but transitions to orifice flow as the ponded depth increases. The following procedure is used for calculating the inlet capacity:

    Anchor: #LFAHJYTP
  1. Choose a grate of standard dimensions to use as a basis for calculations.
    2. Anchor: #BQBEPYWQ
  2. Determine an allowable head (h) for the inlet location. For a grate in a curb and gutter section, this should be the lower of the curb height or the depth associated with the allowable ponded width. For a grate in a ditch (drop inlet), this should be the lower of the height of the ditch block, if any, or the allowable ponded depth. No gutter depression is applied at grate inlets.
    3. Anchor: #OMPOSDNG
  3. Determine the capacity of a grate inlet operating as a weir. Under weir conditions, the grate perimeter controls the capacity. Figure 10-16 shows the perimeter length for a grate inlet located next to and away from a curb. The capacity of a grate inlet operating as a weir is determined using Equation 10-30.

    Anchor: #DFBEUOSX

    Equation 10-30.

    where:

      Anchor: #IOPVSBWN
    • QW = weir capacity of grate (cfs or m3/s)
      • Anchor: #DGYDQXSJ
    • CW = weir coefficient = 3 for English measurement or 1.66 for metric
      • Anchor: #SYCHCQMV
    • P = perimeter of the grate (ft. or m) as shown in Figure 10-16: A multiplier of about 0.5 is recommended to be applied to the measured perimeter as a safety factor.
      • Anchor: #QMNAQPMK
    • h = allowable head on grate (ft. or m).

    Perimeter Length for Grate Inlet in Sag Configuration (click in image to see full-size image) Anchor: #BKCHKOFYgrtop

    Figure 10-19. Perimeter Length for Grate Inlet in Sag Configuration

    4. Anchor: #NWUQKNNL
  4. Determine the capacity of a grate inlet operating under orifice flow. Under orifice conditions, the grate area controls the capacity. The capacity of a grate inlet operating under orifice flow is computed with Equation 10-31.

    Anchor: #URARORNW

    Equation 10-31.

    where:

      Anchor: #CRYXBDXB
    • Qo = orifice capacity of grate (cfs or m3/s)
      • Anchor: #ULIRGFGM
    • Co = orifice flow coefficient = 0.67
      • Anchor: #TAGGUTQD
    • A = clear opening area (sq. ft. or m2) of the grate (the total area available for flow). A multiplier of about 0.5 is recommended to be applied to the measured area as a safety factor
      • Anchor: #NPATWQII
    • g = acceleration due to gravity (32.2 ft/s2 or 9.81 m/s2)
      • Anchor: #ARTAEDKH
    • h = allowable head on grate (ft. or m).
    5. Anchor: #ISPDAIWV
  5. Compare the calculated capacities from steps 3 and 4 and choose the lower value as the design capacity. The design capacity of a grated inlet in sag is based on the minimum flow calculated from weir and orifice conditions. Figure 10-17 demonstrates the relationship between weir and orifice flow. If Qo is greater than Qw (to the left of the intersection in Figure 10-17), then the designer would use the capacity calculated with the weir equation. If, however, Qo is less than Qw (to the right of the intersection), then the capacity as determined with the orifice equation would be used.

    Relationship between Head and Capacity for Weir and Orifice Flow (click in image to see full-size image) Anchor: #LPNQIWCIgrtop

    Figure 10-20. Relationship between Head and Capacity for Weir and Orifice Flow

Previous page  Next page   Title page